Since f is injective there can be at most a single a such that fa b Also if fa b then gfa a by construction Hence g is a left inverse of f gb. We know that A inverse of a function fx exist if it is both 1-1 and onto A We know that the modulus function is not 1-1.
Fx 3x4 f x2 f x fx2x5.
. This function is called a one-to-one function. 𝑓𝐙 𝐙 𝑓𝑥 𝑥 4 b. Select the function that has a well-defined inverse.
A function f from a set X to a set Y assigns exactly one element of Y to each element of XThe set X is referred to as the domain of f and the set Y is the co-domain of f. Select the function that has a well-defined inverse. F may not have a well-defined inverse ff1IAff-1IA ff1fff-1f ff1IB.
In both the cases we have. Since for all y R f 2 y 3 y f 2 x 3 x 2 x 3 f 1 x f 1 x 2 x 3 Hence the required f 1 is given by f 1 x 2 x 3. Therefore it is possible but not necessarily true that f is onto.
Which of the following is the inverse of the given function. Click again to see term. Y x - 13.
Well contradiction is a reasonable method to attempt. Tap again to see term. The domain and the range of mx are all real numbers as well as the domain and the range of.
This line in the graph passes through the origin and has slope value 1. Each choice defines a function whose domain is A and whose target is X. 𝑓𝐙 𝐙 𝑓𝑥 𝑥2.
A and B are finite sets. The domain of f is larger than the. If f is a one-to-one continuous function defined on an interval then its inverse function f-1x is also continuous Derivative of an Inverse Function f-1a 1ff-1a.
Select the true statement. Since there are two different x such that the function have the same value. The domain of f is at least as large as its target.
𝑓𝐙 𝐙 𝑓𝑥 2𝑥 5 c. Hence the function has an well defined inverse. The function f has a target with 4 elements and a domain with 8 elements.
If f is one-to-one then the target must be at least as large as the domainYes No 2 Is it possible that f is onto. Click again to see term. A common addendum to a formula defining a function in mathematical texts is it remains to be shown that the function is well defined For many beginning students of mathematics and technical fields the reason why we sometimes have to check well-definedness while in other cases we dont remains elusive.
Its a useful exercise to show that if f has an inverse g then g is unique that is if h is also an inverse of f then hg here use the definition of the identity functions to prove this. Computer Science questions and answers. As we know for a function fAB to be well-defined every element of aA must be mapped with any element of BInverse function - if f Page 8 of 9 33 Select the expression that is equal to 3 k1 2 a 3 k2 b 3 k3 c 3 2k1 d 3 2k2.
The function that has an inverse function is. Now we will find the inverse of f. The function fAB is a bijection.
It has a left inverse Proof. 𝑓𝐙 𝐙 𝑓𝑥 𝑥 d. Functions bx and px are not one-to-one functions see attached diagram then you cant find an inverse function.
The graph of the inverse of a function reflects two things one is the function and second is the inverse of the function over the line y x. Tap card to see definition. And take x 7.
Click card to see definition. Yes No 3 Is it possible that f is a bijection. Tap card to see definition.
Select the true statement. QuestionSelect the function that has a well-defined inverse. A B is a bijection.
A f f-1 f b f f-1 IB c f f-1 IA d f may not have a well-defined inverse. Now how can we show that if fcolon Ato B is invertible then f must be a bijection. A and B are finite sets.
Function dx doesnt include x then you cant also find an inverse function. A B is injective Pick any a 0 in A and define g as a if fa b a 0 otherwise This is a well-defined function. If the inverse of a function is itself then it is known as inverse function denoted by f-1 x.
A f a 3 b 4 c 3 d 4 b f a 3 b 3 c 3 d 3 c f a 3 b 4 c 2 d 1 d f a 3 b 4 c 2 d 4 33 Select the expression that is equal to 3k12 a 3k2 b k33. Select the function that does not have a well- defined inverse.

Inverse Trigonometric Functions Class 12 Notes Maths Chapter 2 3 Trigonometric Functions Class 12 Maths Math

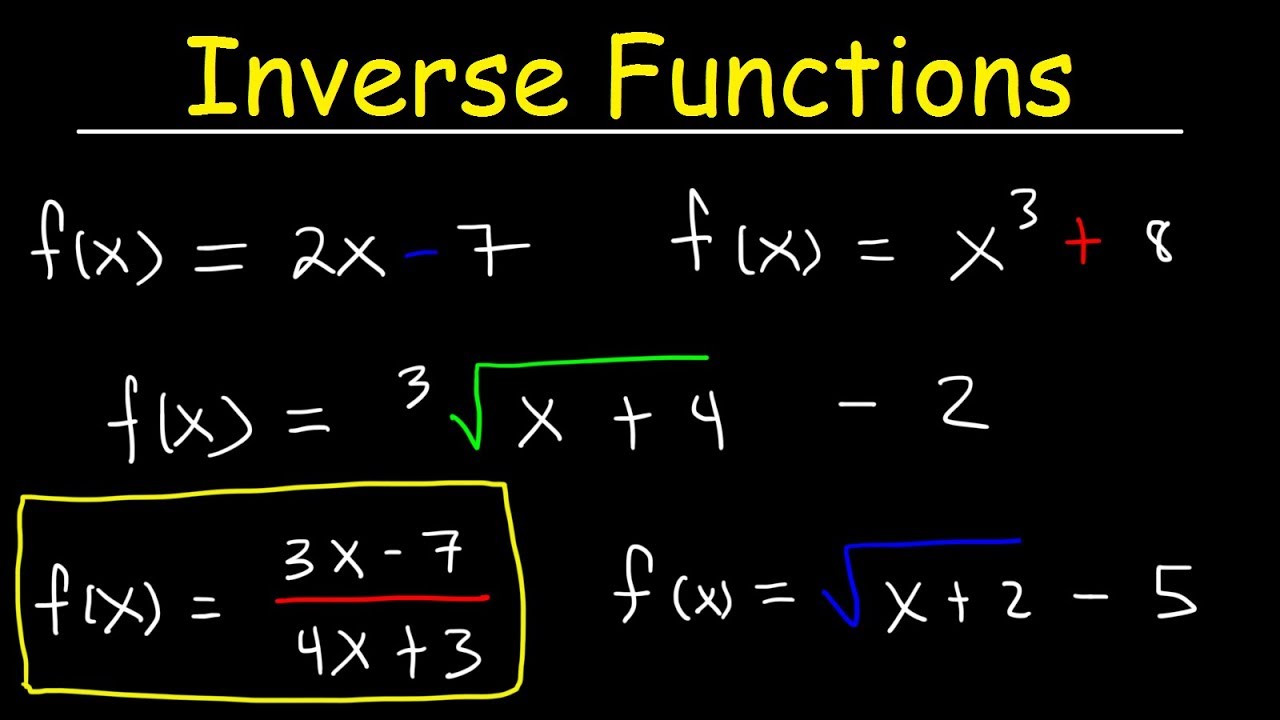
Inverse Functions Domain Range With Fractions Square Roots Graphs Youtube
0 Comments